Cultural-Historical Psychology
2023. Vol. 19, no. 4, 46–55
doi:10.17759/chp.2023190405
ISSN: 1816-5435 / 2224-8935 (online)
Exploring Paradoxes in the Development of Mathematical Thinking: a Cultural-historical Perspective
Abstract
Primary school teachers are the educators responsible for introducing children into the world of mathematics. In the process of learning mathematics, children may experience negative emotions and develop negative attitudes or mathematics anxiety. Some authors approach these issues by focusing on primary teachers’ mathematics anxiety or proficiency in mathematics. In this article, we explore whether and how certain social and educational practices shape or not the development of mathematical thinking in prospective primary teachers, from a cultural-historical perspective. The study’s sample consists of twelve prospective primary teachers in their last year of studies. The participants were interviewed about their experience with mathematics throughout their educational years. By examining and contrasting the different developmental trajectories of the participants, we identified their “imprisonment” in situational thinking and some of the paradoxes that characterise their development. Adopting a historical perspective in analysing participants’ trajectories was crucial in uncovering the paradoxical nature of the development of mathematical thinking. We conclude by highlighting the socially mediated nature of mathematics and the obstacles posed by the competitive and exam-driven nature of educational practices
General Information
Keywords: development of mathematical thinking, prospective primary teachers, situational thinking, conceptual thinking, paradoxes
Journal rubric: Theory and Methodology
Article type: scientific article
DOI: https://doi.org/10.17759/chp.2023190405
Funding. The reported study was funded by the University of Crete’s Funding Committee (decision number: 538/15-11-2021)
Received: 23.10.2023
Accepted:
For citation: Anastasakis M., Dafermos M. Exploring Paradoxes in the Development of Mathematical Thinking: a Cultural-historical Perspective. Kul'turno-istoricheskaya psikhologiya = Cultural-Historical Psychology, 2023. Vol. 19, no. 4, pp. 46–55. DOI: 10.17759/chp.2023190405.
Full text
Introduction
Many of us have told or heard stories about negative experiences of mathematics at school; expressions such as “I hate mathematics” or “I had a really bad math teacher” are not only common but they actually are quite older than we think. For example, consider the following Elizabethan verse:
Multiplication is my vexation
And division is quite as bad
The Golden Rule is my stumbling-stool
And Practice drives me mad[1].
In this text from the 1570s, the unknown author has successfully captured the negative emotions that many 21st century students experience when learning mathematics. So, one may reasonably ask: why is this the case? Why mathematics, despite of the advances in science and the overall societal changes that have occurred over the last 500 years, can still elicit such strong emotions? At the following we discuss representative findings from two main sources of the scientific discourse; the literature on mathematics anxiety (MA) and primary teachers’ proficiency in mathematics.
MA is the feeling of fear and tension that a person experience when manipulating numbers or solving a mathematical problem [17]. Some authors treat MA as a personal characteristic pertaining to difficulties with numerical and spatial processing [13], gender [11], general anxiety [10] or genetic predispositions [22]. For others, MA is linked to socio-environmental factors such as negative experiences at home or in the classroom [2; 16]. In the case of the latter, a lot of attention has been drawn to the role of primary teachers who engage in dogmatic teaching practices [3; 14], overemphasise rote learning [20] or instil gender-stereotyped beliefs [4].
Another dimension that has been widely discussed in the literature pertains to the mathematical competence of primary teachers. A growing body of research suggests that a mere proficiency in mathematics alone is not sufficient and that primary teachers need to develop a deeper understanding of mathematics [9]. One of the most influential approaches in this area is Shulman’s [18] distinction between content knowledge (the knowledge related to a particular subject) and pedagogical content knowledge (a combination of content and pedagogical knowledge). Although Shulman’s approach has been criticised [8], the focus on content is evident in various policy documents that seek to answer questions such as “What should future primary teachers know about mathematics?” [see 5 for some examples]. In our view, this perspective has created a dichotomy that is reflected in many teacher preparation courses: the university curriculum is divided between modules that focus on either mathematics or on how mathematics should be taught.
Furthermore, current views of the affective and the knowledge domains fail to capture the issues surrounding primary teacher education because they do not adopt an approach that pays attention to the development of mathematical thinking. Among the few attempts on that front is Simon’s [19] concept of key developmental understanding, a construct that highlights significant milestones in a student’s mathematical development and is based on Piaget’s [15] notion of reflective abstraction.
It seems that the issues surrounding the unpreparedness of primary teachers in mathematics require a developmental perspective, which is currently lacking in the literature. Questions such as “do primary teachers have MA because of their reduced math abilities?” or “what kind of knowledge do future primary teachers need?” miss the point and focus on phenomenological aspects rather than on the underlying mechanisms that give rise to such phenomena. In our view, a more meaningful approach to unravel this issue would be to look at the development of primary teachers’ mathematical thinking. Consequently, in this paper we ask: How does the nature of mathematics teaching and learning contribute or not to the development of mathematical thinking in prospective primary teachers?
Theoretical framework
Vygotsky used the term ‘obuchenie’ which refers to a two-way process of teaching and learning. The problem of the relationship between obuchenie and development was examined by Vygotsky as “the most central and fundamental question” [21, p. 3]. For Vygotsky, the relationship between ‘obuchenie’ and development is dialectical: on the one hand, learning is based on a certain level of cognitive development, and on the other, learning plays a guiding role in development.
The relationship between ‘obuchenie’ and development is not static but is influenced by the organisation of society and the educational system. It is important to underline that not all forms of instruction necessarily lead to meaningful cognitive development. “No one has ever argued that teaching someone to ride a bicycle, to swim, or play golf (forms of activity that are much more complex than the discrimination of the magnitude of angles) has any significant influence on the general development of the child's mind” [21, p. 200].
The transition from situational thinking to the level of conceptual thinking is an important dimension of cognitive development. Situational thinking relies primarily on sensory perception focusing on understanding and responding to the immediate context or situation at hand. In contrast, conceptual thinking involves the ability to generalise, and think beyond the immediate context [12]. Theoretical generalisation as an integral part of conceptual thinking involves the ability to go beyond superficial observations to understand the deeper principles and relationships underlying the phenomena being studied [6; 7].
The dominance of empiricism and narrow pragmatism in the classroom can inhibit the transition from visual-effective and concrete-pictorial thinking to theoretical thinking, thus hindering the development of higher mental processes. If instructional practices prioritise only empirical observation and practical application, students may struggle to engage in theoretical reasoning, problem solving and critical thinking, which are crucial for cognitive development.
Methodology
Context, Participants and Data Collection
In Greece, students are admitted to a university after passing national qualifying examinations (Panhellenic Exams). Students wishing to become primary school teachers can choose a route that may or may not include mathematics as an examination subject. Therefore, it is very common for prospective primary teachers to have a two-to-three-years gap in studying mathematics when they enter the university.
The study took place in a Department of Primary Education in Greece and was approved by the university's research ethics committee (decision number: 27/24.02.2022). Our sample consists of 12 undergraduates (prospective primary teachers) who were interviewed during the spring semester of 2022 (average age: 21.3 years, 91.6% females). Due to COVID-19 related restrictions, all interviews were conducted via Zoom. The interviews were semi-structured and each session lasted an average of 40 minutes. No incentives or other types of compensation were offered to participants.
The interview protocol included open-ended questions focusing on four topics (Table 1). Although the question “How would you describe your relationship with mathematics?” was intended to be used as an “icebreaker”, it proved to be the one that allowed both the participants and the authors to unlock a developmental perspective i.e., the interviews became “life stories”.
Table 1. The interview protocol questions
|
Topic |
Question |
|
Overall experience with mathematics |
How would you describe your relationship with mathematics? |
|
Events and difficulties |
What kind of difficulties did you encounter in school/ at the university? Can you recall any particular event? |
|
Emotions |
How did you feel when this happened? |
|
Coping |
Did you try to overcome these difficulties? How? |
Analytical approach
All the interviews were transcribed verbatim. Initially, both authors spent time in reading the transcripts in both literally and interpretatively. They then engaged independently in an open coding process; the codes were then brought together and discussed. The main focus of this process was not to reach agreement but rather to gain an integrative understanding in terms of both complementary and conflicting views. The codes were then combined and organised into thematically related groups (themes).
During this stage, it became clear that some participants had serious problems with mathematics while others did not; this observation was crucial for the subsequent analysis, as the approach described above was not sufficient to capture the diversity found in the data; therefore, the interviews were divided into two groups. A summary was then written for each case and the themes covered in each interview were collected. Finally, all the summaries were tabulated and the two groups were compared.
Findings
In the following sections we first describe the main characteristics of the two groups and the themes identified in the interview data. We then compare the themes between the two groups and finally, we introduce and present a representative case for each group.
Description of the two groups
Using the participants’ accounts of their experiences with mathematics as a criterion, we created two groups, group A and group B. Participants in group A (n=6), reported having received low grades throughout their schooling years, having experienced constant serious difficulties in learning mathematics, and reported having developed negative attitudes towards mathematics or even MA. In contrast, participants in group B (n=6) had an unproblematic experience with mathematics; they reported having good grades throughout their schooling years and conveyed a positive attitude towards mathematics. Extracts from the interviews illustrating these differences are presented in Table 2.
Table 2. Participants’ description of their relationship with mathematics
|
Group A |
“My relationship with mathematics was not good” (S01) |
|
“I was always afraid of mathematics” (S04) |
|
|
“I always felt inadequate in this subject” (S06) |
|
|
“I started doing again mathematics at the university because I had to” (S07) |
|
|
“My relationship with mathematics was strange, I always felt anxious” (S08) |
|
|
“My relationship with mathematics was never good” (S12) |
|
|
Group B |
“I love mathematics since a very young age” (S02) |
|
“In general, I like mathematics” (S03) |
|
|
“I have a very good relationship with mathematics” (S05) |
|
|
“I reached a point that I started to love mathematics” (S09) |
|
|
“I was always a very good student; all subjects were the same for me” (S10) |
|
|
“I was very good at school and I liked mathematics” (S11) |
Themes
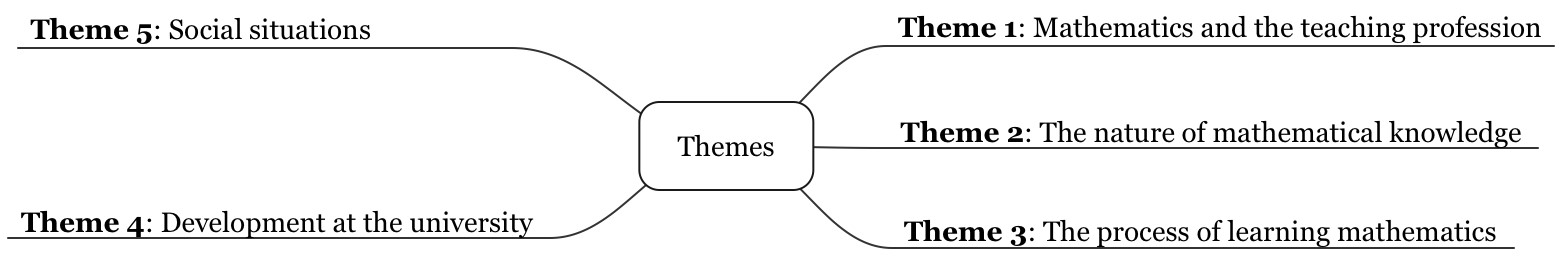
The analysis led to the identification of five themes that were common to all cases (Figure 1): theme 1 captures participants’ views about the kind of knowledge that primary teachers should learn at the university in order to be able to teach mathematics in schools; theme 2 pertains to participants’ opinions about a person’s ability to learn mathematics; theme 3 expresses participants’ views on the approach and types of procedures involved in learning mathematics; theme 4 represents participants’ views and self-reflections about the development of their mathematical thinking at the university and; theme 5 summarises the social situations that participants recall having experienced while learning mathematics throughout their formal education.
Fig.1. The five themes identified in the interview data
Comparisons between groups
By comparing and contrasting the themes between the groups, we identified whether and how the participants’ views converged or diverged (Table 3). With regard to theme 1, both groups expressed the view that the teaching and learning of mathematics at the university should replicate the school curriculum. In relation to theme 2, participants from group A considered mathematics to be an innate ability, a process that has an end. On the contrary, the participants of group B described mathematics as a way of thinking that is governed by its own laws, a kind of knowledge that can be cultivated and should be approached as a developmental process. In relation to theme 3, both groups described their approaches to learning mathematics in an exam-driven way, a process that requires minimal effort. With regard to theme 4, both groups expressed the view that virtually nothing significant had been learned at the university. Finally, regarding theme 5, participants from group A reported traumatic events (some at an early age), whereas participants in group B reported insignificant social situations or no traumatic events at all.
Table 3. Theme comparisons between groups
|
Theme |
Group A |
Group B |
|
(1) Mathematics and the teaching profession |
The teaching-learning of mathematics at the university should replicate the school curriculum. |
|
|
(2) Nature of mathematical knowledge |
Understanding mathematics is an innate ability |
Understanding mathematics is a developmental process |
|
(3) The process of learning mathematics |
Learning requires minimal effort |
|
|
(4) Development at the university |
Absence of development at the university |
|
|
(5) Social situations |
Traumatic events |
No events/minor events |
Representative cases
Group A: Penelope
Penelope was in her fourth year at university when she was interviewed and was currently doing her practicum in a public school. Penelope’s developmental trajectory was characterized by a gradual disengagement from mathematics that began in primary school and intensified through secondary school. At the beginning of our interview, Penelope described her overall experience of mathematics as follows:
In primary school... my relationship with mathematics was mediocre... I didn't do badly it was just… I always felt at a disadvantage in mathematics when compared to other subjects... In junior high school [Gymnasium] it got worse, I wasn’t doing well at all… in senior high school [Lyceum] I had distanced myself, I didn't pay attention, I pretended that mathematics didn't exist because they caused me anxiety... then at the university mathematics was a necessary evil so to speak...
Penelope’s rite of passage into the world of mathematics was an unpleasant encounter, an experience marked by a series of dramatic events involving her primary school teacher. According to Penelope, the root cause of her unsuccessful journey could be traced to her teacher's general classroom behaviour (e.g., being strict) and pedagogical approach (e.g., following the book to the letter), an attitude that caused Penelope to experience a range of negative emotions that led her to develop MA:
We had a teacher somewhere in fourth/fifth/sixth [grade] somewhere around there… […] this is where I kind of lost it, because she didn't treat us nicely in mathematics... to anyone who didn't understand.... she kept calling the children up to the blackboard and… when I was on the blackboard... that caused me a lot of anxiety because... I felt that all my classmates were looking at me, how I would solve the problem… the teacher was quite demanding... and since then I think I had... I have had quite a hard time with mathematics...
During junior high school, Penelope’s relationship with mathematics deteriorated even further due to a mathematics teacher who showed no particular interest in supporting his students. This led Penelope to develop an apathy towards mathematics, which gradually led her to totally distance herself in relation to mathematics:
In junior high school we had a completely indifferent teacher... he never explained the mathematical concepts or theory to us…. we just went in and out of the class... and then we did tests... apparently nobody did well at all, this was reflected in my grades, then it became bigger and... I gave up at some point...
Although Penelope’s developmental trajectory was characterized by disruptions and discontinuities, when asked if and how she was coping with her situation, her response indicated an intention not to deal with her gaps properly but to rather “fix” them temporarily, a choice legitimized by her teacher’s attitude:
I only had private lessons for a while in junior high school... one summer... and I think it went well but when the schools opened again and I saw the teacher's indifference, I automatically became disinterested in the class... and since we only had 15 subjects, I decided to study the rest [and not mathematics] ...
Penelope’s learning philosophy became more evident when she was asked to reflect on her experience of mathematics and her approach in dealing with difficulties faced during her school years:
At first, I was trying to cope with it, I was trying at home as much as I could, studying, looking for ways to explain it to myself so that I could understand mathematics, so that I could move on, so that I could be good, because... I wanted to be good, I had a very good course throughout the school years and it annoyed me that I was behind only in this subject, while I was very good in everything else... And I tried at first at home to explain it with my mom many times, especially in primary school, and then in secondary school on my own, I tried to study mathematics... but I couldn't, it was difficult...
By the end of junior high school and at the beginning of senior high school, Penelope had already decided to choose a direction in her studies that did not include mathematics as a subject. This choice was further confirmed by a self-perception that she was more competent in the humanities rather than in the natural sciences or mathematics:
Yes, I had decided on my major from very early on because I could see that I had an inclination towards most of the theoretical subjects, that I liked them even more... so...
At the university, Penelope studied two types of modules, one focused on the didactic side of mathematics and the other on mathematics per se. In discussing her experience of the latter, Penelope made a clear distinction between the kind of mathematical knowledge that primary teachers need and the knowledge that which is actually taught at the university:
… I think the material he [the lecturer] chooses to teach is completely alien and unnecessary for us who are going to be primary teachers... we could focus on parts of the school curriculum, practice on things, do examples from the school textbook, I don't know... I think it's all a mistake... Why don't we do the things that we are going to teach in primary school?
Penelope’s narrative revealed an instrumental, utilitarian and pragmatic approach to knowledge and learning; for her, the teaching and learning of mathematics at the university should be examined in terms of its narrow application i.e., as a replication of the school curriculum. This view was further elaborated when Penelope described having a relatively unproblematic experience with the mathematics education modules; Penelope felt motivated because she saw a clear connection between the material taught and the school curriculum:
…because we were focusing exactly on the things that we are going to teach later... there was also a motivation to study it because you know it will come in handy...
Penelope’s exposure to dramatic events at an early age, had left an important imprint on her; while discussing about the emotions of fear surrounding her every time she was involved in activities with mathematical content, she commented about her experience in her practicum:
I am currently doing my practicum… I've been asked to teach mathematics both semesters... while it is very easy for me to prepare, study, find activities or alternative ways of teaching in other subjects... in mathematics I don't know why, I can't do it... I feel too stressed… especially with fractions… I think I can't do this thing to myself, every time I'm confronted with fractions...
Group B: Alexandra
Alexandra was in her third year at university when she was interviewed. At the beginning of her journey, Alexandra had severe difficulties with mathematics due to the apathy of her primary teacher; as a result, after finishing primary school, Alexandra had many gaps and gradually developed a negative attitude. At the beginning of junior high school, Alexandra’s struggle continued but her aversion to mathematics slowly disappeared:
…during the first and second year of junior high I struggled a lot, because in primary school I had no basis at all… (Why?) My teacher at the time... he was bored teaching us and he didn't do much... so I didn't get any basis in mathematics and when I started junior high, I got too stressed… I started too abruptly, too much knowledge and I got stressed... and at first, I didn't want that, I didn't like it but after a while... I had a very good math teacher who started to made me love mathematics a bit more...
By the end of junior high, Alexandra had developed a healthy relationship with mathematics and she began to enjoy it. During senior high school, Alexandra’s attitude towards mathematics underwent a transformation; she felt exposed to a world governed by logic, a language that required from the person practising it to understand its principles and develop a method for applying them when solving problems:
...in senior high, I started to love mathematics… I liked that it was... simple logic that required to develop a method in your head to make it work out in order to do what you want to do... our teacher was very nice and he seemed very passionate about what he was doing and he was happy to teach us and that was evident... and he showed us a little bit of, let's say, the magic of mathematics...
The above demonstrate that Alexandra’s developmental trajectory during primary and secondary education was not characterized by dramatic situations or incidents with irreversible consequences. However, Alexandra’s exposure to mathematics in junior and senior high school seems to have been of significant developmental importance.
Since senior high school, Alexandra has wanted to study physics and after passing the Panhellenic Exams, she enrolled as a physics major. However, the demanding nature of the course and Alexandra’s love of working with children, led her in taking the decision to change major:
…although I really liked physics and its logic... the study of physics required people who liked only physics… and I was a bit more artistic, especially with the children, I had a very big fondness and... I believe very strongly in the educational values... so, I changed my major...
At university, like all prospective primary teachers Alexandra took modules with a purely mathematical content and modules that focused more on the pedagogical aspects of teaching mathematics. Given her love of mathematics, she was excited to attend modules from the first category and chose Geometry, a module that all of her fellow students avoided. It was at this point that, Alexandra realized that, unlike her, the majority of undergraduates did not have the necessary mathematical background. In discussing her overall experience with these two types of modules, Alexandra made a distinction in terms of their usefulness:
The truth is that the two compulsory modules in mathematics education… I don’t think they will help me very much in teaching mathematics to children, the content was a bit... it wasn’t for me... (Was it boring?) quite... we were taught games which… I understand their use... that it is important to learn how to use these games too but… it was not something... to show children how to love mathematics, why mathematics is needed in everyday life and… a little bit more complicated things, the module’s content was very basic… and I understand that even for these modules, I saw... my fellow students straining too much...
While discussing her experience with these modules, Alexandra described them as “boring” and admitted that she only attended lectures to have access to the exercises solved by the lecturer:
…there were times when… I put my headphones on [listening to music] and just noted down what he was writing on the board which was... very basic… I didn't even have to listen... in the end, the only reason I went to the class was because he [the lecturer] was solving problems that he didn't upload them somewhere else afterwards... and I couldn't get them from anybody else...
Although Alexandra saw mathematics as a way of thinking that is cultivated, at a later stage of the interview she saw her ability to formulate her thoughts in writing as having an innate character and contrasted this with her background in physics and mathematics:
I see in myself a difficulty in writing long texts in assignments, because... while I have done a report like we all did in high school, I don't know, maybe it's because I have a science background and I've learned to think like that? That I too have not cultivated it enough? eh... it's not so easy for me to write long texts, that is, I have a problem with written expression not oral, it's a bit difficult for me to write...
Discussion
Four out of the five themes identified in the data are manifestations of the main mechanism that has caused disruptions and discontinuities in the participants’ development: situational thinking. Views about mathematics and its relationship to the teaching profession (theme 1), the nature of mathematical knowledge (theme 2), and the process of learning mathematics (theme 3), reveal participants’ exposure to and internalisation of forms that promote narrow and dualistic views of mathematics. At the university, the consequences of situational thinking are observed, as well as its ongoing work in further corroding and damaging the development of undergraduates who will eventually become primary teachers (theme 4). Although our data could not support further analysis and interpretation, the traumatic experiences reported by some participants (theme 5) suggest that additional, non-cognitive mechanisms are also in play and highlight the significant role of emotions in the development of mathematical thinking. Below we unpack the role of situational thinking and further emphasise the complex, dynamic and dialectical nature of development by presenting paradoxes revealed by the juxtaposition of conventional views about “success” and conflicting views of the mediating role of educators.
Situational thinking
The participants’ situational thinking was captured by themes 1-4 in the form of (a) an avoidance of complex ways of thinking; (b) a nativist view of mathematics and; (c) an emphasis on operations and procedural knowledge. The limited view of the nature, function and value of the mathematical knowledge that is necessary for primary teachers (theme 1) was expressed by both groups and displays an avoidance of more complex, theoretical ways of thinking. All participants saw the content of mathematics taught at the university as an advanced and unnecessary kind of knowledge, whereas those in group B regarded the didactic side of mathematics as a body of knowledge that is separated and irrelevant to the teaching and learning of mathematics. Regarding the epistemological nature of mathematics (theme 2), participants from group A saw mathematics as an innate endowment, a capacity that only some of us are born with. On the contrary, participants from group B regarded mathematics as a way of thinking that can be nurtured, a process that takes time.
With regard to the learning process (theme 3), all participants adopted an exam-driven approach and reported employing strategies such as memorising theorems and proofs or attending lectures just to have access to problems solved by a lecturer. They described a learning culture that demands immediacy and rejects the effort required to engage meaningfully with mathematics. This approach to learning emphasises operations and procedural knowledge because it shows that participants’ focus on the steps required to achieve a goal (either at a micro or a macro level) rather than on a deep understanding of the abstract and general principles of mathematics.
The development of mathematical thinking at the university (theme 4) was a task that remained unrealised for the majority of participants. This was the result of a curriculum that reinforced participants’ situational thinking and failed to introduce them to more advanced, theoretical forms of thinking. The participants of group A felt motivated by the practical orientation of some modules’ and were repelled by modules that dealt with mathematical content that goes beyond the school curriculum. On the contrary, all participants of group B felt bored and demotivated by modules focusing on “simple” mathematics and most of them preferred to focus on modules with advanced mathematical content.
Given that the transition from situational to conceptual thinking requires fundamental changes in the types of activities with which subjects are engaged [12], it can be argued that throughout their education (primary, secondary, tertiary) the participants were not exposed to activities that could facilitate this transition. This reveals not only the participants’ current “imprisonment” in ways of thinking internalised at earlier stages of their development stage but also their continued “imprisonment” in mandatory teaching and learning activities that have been proved to be detrimental to their development. As a result, many participants view knowledge in a static way: they hold the erroneous view that knowledge acquired at an earlier developmental stage (e.g., mathematics learned at secondary school) has the same potential at a present time (e.g., mathematics needed at the university and to become a primary teacher).
Paradoxes
When success becomes a failure and failure becomes a success
As mentioned above, participants in group A have had experienced severe issues and a constant failure with mathematics whereas, participants from group B had encountered minimum or no difficulties at all and have had progressed successfully throughout their education. In this sense, one might expect that the same pattern would continue at the university; however, a closer look at two cases demonstrates the exact opposite, i.e., that students who have always struggled with mathematics may actually make qualitative leaps and transformations during their time at university whereas, students who have done well may not develop at all.
Daphne (participant S07, group A) had a problematic trajectory with mathematics since primary school and was obliged to study mathematics again at the university. When Daphne began to describe her experiences during the first year of her studies, she referred to a crisis: becoming a teacher requires the ability to transform mathematical knowledge into meaningful activities and exercises that would support the teaching and learning of mathematics in a class. For Daphne, this change was more challenging than revising the mathematics behind each exercise because it involved a shift from consuming knowledge to applying knowledge and producing new knowledge in the form of a new exercise or activity.
It was... a bit strange because for me mathematics was equations, it was geometry, it was exercises... at the university it was a bit more about the methodology of mathematics and how to learn to think about the reasoning behind each exercise... because we wouldn't solve them, the children at school would... and it was a bit difficult to learn to think like that… (Which was more difficult?) It was more difficult to prepare something for the students. Knowing how to solve the exercises was difficult at first, but after a while it went well…
Christos (participant S10, group B) described himself as an “excellent student” and mentioned that he had consistently high grades in all subjects throughout his schooling years. At the university, Christos mentioned on two occasions that he had problems with mathematics. The first relates to a module in Geometry; Christos solved the exam paper by relying on the mathematical knowledge he had acquired in secondary school and was expecting his grade to be 10 but he was instead marked with a 7.5 (on a scale of 10). When Christos asked the lecturer about his grade, the lecturer replied that he should have used the methods and tools demonstrated in the lectures:
It was unfair… as long as the problems were solved correctly, there is no reason to be selective when marking, I mean, personally I don't think it's right to... it’s unfair in my opinion to a student, although I don't mind if... I got 7.5 or 10, I'm examining this… from a philosophical point of view, I don't think it was fair…
The second occasion relates to a module on probability and statistics. This was the first time Christos had been taught statistics at the university and he found it very difficult to understand this part of the curriculum. He described the module as challenging and questioned the value of statistics in relation to the teacher profession.
I'm doing statistics for the first time; we did not have this subject at school… but… I don’t know… in what way this kind of knowledge would be useful to me... this kind of mathematics makes me say, "I'm a teacher, do I really need this?”
Nature or nurture?
In the interviews, we became aware of the echoes that characterise the everlasting nature-nurture controversy: participants in group A took a nativist position on the nature of mathematical knowledge, whereas those from group B regarded mathematics as a developmental process. However, a closer examination of some of the cases draws our attention to an oxymoron: although participants from group A saw mathematics as an innate ability, they simultaneously considered teachers as the most crucial resource in understanding or not mathematics; similarly, while participants from group B regarded mathematical knowledge as a way of thinking that could be cultivated, they also rejected the mediating role of educators by negating any kind of knowledge associated with the teaching praxis. We illustrate the above by presenting extracts from two cases.
Thalia (participant S08, group A) described mathematics as a subject that always caused her anxiety and fear; she attributed her problematic experiences to teachers who were never able to properly support her learning in mathematics. Thalia recalled a dramatic event that happened in junior high school involving her maths teacher:
in the third grade’s exams, I did badly in mathematics, although in all the other subjects my grades were perfect... I had studied so much… and I accidentally came across my teacher on the street and he told me... “you know, I didn't expect this from you” and he added “you'll have to work on it, now that you're entering senior high, especially if you want to follow this direction in your studies”... after that, I knew that no matter how well prepared I am in mathematics, I would always have this insecurity...
Thalia attributed this insecurity to her inability to work independently; as she elaborated on this, she indirectly highlighted the fundamental role of an educator in extending her current stage of development to autonomous forms of learning:
…it was stressful for me to solve problems without someone telling me “This is right" and “keep going”... when my teachers were present and I could show them my solution... if there was a mistake somewhere they could tell me “Oops that's wrong” and I would correct it… However, when I solved exercises on my own… I got stuck... while in the other subjects I had no issues at all...
When she was asked to reflect on the root of her insecurity in mathematics, Thalia rejected the role of teachers, dramatic events or other mechanisms that had potentially shaped her development and plainly invoked her innate inclination towards other kinds of knowledge:
No, I just think I'm more of a theoretical mind... that is, I like talking and writing reports more than solving exercises...
Alcestis (participant S02, group B) described mathematics as a way of thinking and expressed her love and deep affection for this subject. Alcestis’ relationship with mathematics was mediated by her parents who introduced her into a new world by playing mathematical games since preschool:
I love math from a very young age... before I went to school, my parents and I used to play math games... I really like the fact that I am now at an age where I can easily solve everyday problems, not just math problems... so… there's a way of thinking behind maths that I really like...
Alcestis described her experience at the university with modules focusing on the didactic side of mathematics as boring and monotonous. According to Alcestis, this was a result of the weak mathematical background of her fellow undergraduates, a problem that forced the lecturer to spend time on “basic” mathematics instead of focusing on the module’s content. Alcestis felt that these lectures were a waste of time and began to skip classes, causing her grades to drop:
The only motivation I had was to get marks... that made me say "stop, I have to attend the lectures in order to pass the module with a good grade" otherwise nothing, I mean I didn't learn anything new...
This lack of interest was the only obstacle Alcestis faced with mathematics at the university. Ironically, although she used the undergraduate population’s lack of mathematical background and grades as a vehicle to justify her exam-driven approach, she acknowledged the mediating role of the lecturer:
I didn't attend the lectures because I knew from the beginning that I was going to be bored... so, I had to study by using only the textbook… it was difficult for me... it wasn't hard it just wasn't the same as being in the lecture and “getting it” from the lecturer...
Conclusion
Far from being an isolated, purely individual endeavour, mathematical thinking is deeply embedded in the wider socio-educational context. At the micro level, the developmental trajectories of individuals in the acquisition of mathematical knowledge are profoundly influenced and shaped by the prevailing social and educational practices. Approaching the development of mathematical thinking as a socially mediated process reveals the intricate complexities and paradoxes inherent in this cognitive journey. This perspective highlights the paradoxes inherent in how individuals engage with mathematical thinking, particularly in the context of the social and educational practices in which they participate. One of these paradoxes relates to the competitive and exam-driven nature of these practices [1]: despite their differences in performance, participants from the two groups converge both in terms of both objective (e.g., denial of alternative or more advanced forms of knowledge) and subjective (e.g., the perception that no new knowledge has been acquired) indicators of their development.
It also highlights how the difficulties in the transition from situational to conceptual thinking in the realm of mathematics are interrelated, among other things, with the wider educational and social practices. The highly competitive and examination-oriented educational system, coupled with the prevalence of narrow utilitarianism and empiricism in both formal and informal curricula, forms a substantial barrier to the development of mathematical thinking. The examination-oriented educational system discourages the promotion of a deeper understanding of mathematical concepts and the development of mathematical thinking. In parallel, the utilitarianism in mathematics education, which emphasizes practicality and real-world applications, can inadvertently restrict students from exploring the creative and theoretical dimensions of mathematics and hinder the transition from situational to conceptual thinking.
[1] “Golden Rule” refers to the rule of three, “practice” refers to financial arithmetic.
References
- Anastasakis M., Robinson C.L., Lerman S. Links between students’ goals and their choice of educational resources in undergraduate mathematics. Teaching Mathematics and its Applications: An International Journal of the IMA, 2017. Vol. 36, no. 2, pp. 67-80. DOI:10.1093/teamat/hrx003
- Ashcraft M.H. Models of math anxiety. In I. Mammarella, S. Caviola, & A. Dowker (Eds.), Mathematics Anxiety: What is known and what is still to be understood. London and New York: Routledge, 2019, pp. 1-19
- Ball D.L. Breaking with experience in learning to teach mathematics: The role of a preservice methods course. Learning of Mathematics, 1990. Vol. 10, no. 2, pp. 10–16
- Beilock S.L., Gunderson E.A., Ramirez G., Levine, S.C. Female teachers’ math anxiety affects girls’ math achievement. Proceedings of the National Academy of Sciences, 2010. Vol. 107, pp. 1860–1863. DOI:10.1037/e634112013-097
- Chapin S.H., Gibbons L.K., Feldman Z., Callis L.K., Salinas A. The Elementary Mathematics Project: Supporting Preservice Teachers’ Content Knowledge for Teaching Mathematics. In Y. Li, R. Howe, J. Lewis, & J. Madden (Eds.), Advances in STEM Education: Developing Mathematical Proficiency for Elementary Instruction. Cham: Springer International Publishing, 2021, pp. 89-113. DOI:10.1007/978-3-030-68956-8_4
- Dafermos M. Developing a Dialectical Understanding of Generalization: An Unfinalized Dialogue Between Vygotsky and Davydov. In C. Højholt & E. Schraube (Eds.), Subjectivity and Knowledge - Generalization in the Psychological Study of Everyday Life. New York: Springer, 2019, pp. 61-78
- Davydov V.V. (1990). Types of generalization in instruction: Logical and psychological problems in the structuring of school curricula. In J. Kilpatrick (Ed.) Soviet studies in mathematics education (Vol 2). Reston, VA: National Council of Teachers of Mathematics, 1990, pp. 1-223
- Depaepe F., Verschaffel L., Kelchtermans G. Pedagogical content knowledge: A systematic review of the way in which the concept has pervaded mathematics educational research. Teaching and Teacher Education, 2013. Vol 34, pp. 12-25. DOI: 10.1016/j.tate.2013.03.001
- Hart L.C., Oesterle S., Swars S.L. The juxtaposition of instructor and student perspectives on mathematics courses for elementary teachers. Educational Studies in Mathematics, 2013. Vol. 83, no. 3, pp. 429-451. DOI:10.1007/s10649-012-9464-0
- Hembree R. The Nature, Effects, and Relief of Mathematics Anxiety. Journal for Research in Mathematics Education, 1990. Vol 21, no. 1, pp. 33-46. DOI:10.2307/749455
- Hopko D.R., Mahadevan R., Bare R.L., Hunt M.K. The Abbreviated Math Anxiety Scale (AMAS). Assessment, 2003. Vol. 10, no. 2, pp. 178-182. DOI:10.1177/1073191103010002008
- Luria A.R. Cognitive development its cultural and social foundations. Cambridge, Massachusetts & London Harvard University Press, 1976. 182 p.
- Ma X., Xu, J. The causal ordering of mathematics anxiety and mathematics achievement: a longitudinal panel analysis. Journal of Adolescence, 2004. Vol. 27, no. 2, pp. 165-179. DOI: 10.1016/j.adolescence.2003.11.003
- Öçal T. ‘I remembered this mathematics course because …’: how unforgettable mathematics experiences of pre-service early childhood teachers are related to their beliefs. International Journal of Mathematical Education in Science and Technology, 2021. Vol. 52, no. 2, pp. 282-298. DOI:10.1080/0020739x.2020.1861349
- Piaget J. Studies in reflecting abstraction. Sussex, England: Psychology Press, 2001. 350 p.
- Ramirez G., Shaw S.T., & Maloney, E.A. Math Anxiety: Past Research, Promising Interventions, and a New Interpretation Framework. Educational Psychologist, 2018. Vol. 53, no. 3, pp. 145-164. DOI:10.1080/00461520.2018.1447384
- Richardson F.C., Suinn R.M. The Mathematics Anxiety Rating Scale: Psychometric data. Journal of Counseling Psychology, 1972. Vol. 19, no. 6, pp. 551-554. DOI:10.1037/h0033456
- Shulman L. Knowledge and Teaching: Foundations of the New Reform. Harvard Educational Review, 1987. Vol. 57, no. 1, pp. 1-23. DOI:10.17763/haer.57.1.j463w79r56455411
- Simon M.A. Key developmental understandings in mathematics: A direction for investigating and establishing learning goals. Mathematical thinking and learning, 2006. Vol. 8, no. 4, pp. 359-371. DOI:10.1207/s15327833mtl0804_1
- Trujillo K.M., Hadfield O.D. Tracing the roots of mathematics anxiety through in-depth interviews with preservice elementary teachers. College Student Journal, 1999. Vol. 33, no. 2, pp. 219-219.
- Vygotsky L.S. Mental development of children in the process of obuchenie. Moscow-Leningrad: Uchpedzig, 1935.
- Wang Z., Hart S.A., Kovas Y., Lukowski S., Soden B., Thompson L.A., . . . Petrill S.A. Who is afraid of math? Two sources of genetic variance for mathematical anxiety. Journal of Child Psychology and Psychiatry, 2014. Vol. 55, no. 9, pp.1056-1064. DOI:10.1111/jcpp.12224
Information About the Authors
Metrics
Views
Total: 225
Previous month: 12
Current month: 4
Downloads
Total: 96
Previous month: 2
Current month: 3