Clinical Psychology and Special Education
2013. Vol. 2, no. 1
ISSN: 2304-0394 (online)
Formation of concept of decimal system in Mexican school children
Abstract
The present study deals with initial formation of concept of decimal system in second year of education at primary school in Mexico (City of Puebla). Our research is based on Activity Theory conception of teaching-learning process and of gradual introduction of scientific concepts in school age. The method has been designed and worked out with the help of actions in which logic, symbolic, spatial and mathematical aspects were implemented. All actions were introduced within divided activity of children in group guided by adult. A pretest-posttest design was used with an experimental group of Mexican school children. The results showed that children have developed the significant skills necessary for understanding the concept of decimal number system. They were also able to apply this concept for new kind if activity al the end of school year. Such new activity was solving of mathematic problems, which was not included in official school program. We consider that proposed method can be an approximation for solution of common difficulties which arise at primary school concerning teaching of mathematics.
General Information
Keywords: school age, skills in mathematics abilities, teaching of numeric system, activity theory applied to education
Journal rubric: Methods and Techniques
Article type: scientific article
Published
For citation: Rosas Rivera, Y., Solovieva, Y., García Flores, M.A., Quintanar, L. (2013). Formation of concept of decimal system in Mexican school children. Clinical Psychology and Special Education, 2(1), Article 6. URL: https://psyjournals.ru/en/journals/cpse/archive/2013_n1/58939 (viewed: 02.07.2025)
© Rosas Rivera Y., Solovieva Y., García Flores M.A., Quintanar L., 2013
License: CC BY-NC 4.0
Full text
Introduction
In Mexico, the way mathematics is thought is a matter of great importance since the level of children’s knowledge in this area is very low (SEP, 2011). This means that pupils of third grade of primary school can only solve exercises with low degree of difficulty (elementary operations, increasing sequence with an additive constant, to identify the predecessor of a number, etc.). The teaching method proposed by Secretariat of Public Education (2011) emphasizes that the teacher's task is to create learning environments in order to make pupils think about the methods that perform, promote communication of mathematical ideas that arise during class and evaluate the level of skills that students develop. Nevertheless, no conceptual knowledge is included in current methodology for mathematics. Secretariat of Public Education only proclaims the necessity of reflection, but there are no real proposals which could support such proposition.
Recent research shows how some kinds of mathematical skills that pupils have developed the first grade. Such skills are: follow a command of verbal and numerical sequence counting process, recognition of the properties of closed numbers (tens, hundreds, thousands units), writing by dictation extended numbers (for example, one thousand five hundred-1000500), reading the carried quantities on numbers segmenting one or two digits and solving of addition problems. All such kinds of operations is achieved without any precise knowledge of decimal number system nor of the formal algorithm. Some studies show that the only strategy used by children in primary school during solution of exercises is counting by fingers, personal drawings and mental calculations (Castaneda, 2008; Buto y Gomez, 2011; Garcia, 2011). It is possible to argue that such level of operations is related to empiric mechanic execution.
The introduction of Decimal System concept represents one of the essential aspects of learning at primary school. This is the theme which occupies most of the activities in the first three years of primary school education (Ruesga y Guimaraes, 2011). The decimal number system is considered a positional system, because the figures are not independent but are subject to their position (Silva y Barela, 2010; Avila y Garcia, 2008; Luria, 1995). Each new column of the decimal number system is considered as a new measure count, which is 10 times greater than the extent of the previous column, for example, 10 units of the first column (units) given unit of the second column (tens). The reflection of such relationships could allow students show arithmetic actions, laws and combination translational. It is also possible to emphasize that counting by equal groups (not necessary by 10 units), allows preparation of the conceptual introduction of multiplication. Subsequently division could be shown to the children as the action contrary to the multiplication. Showing the principles of construction of numbering system makes them able to create new measurements of counting, working with the network of columns of numbers (M, C, D, U). Continuation of this logic permits to introduce fractions and decimal fractions at the next step (Talizina, 2009, Salmina, 2001).
The Activity Theory applied to the process of school education (Talizina, Solovieva and Quintanar, 2010; Solovieva and Quintanar; 2010; Galperin, 2009; Talizina, 2009; Salmina, 2001) shows that in order to allow children to assimilate and generalize academic content, teaching should be done through the following stages: 1) stage of motivation, 2) guide based on the action, 3) material-materialized stage, 4) perceptual stage, 5) external speech stage, 6) the internal language stage. Specific types of such actions should be proposed for assimilation of Decimal System concept. Specifically, mathematic content is organized around four components: logical, symbolic, mathematical and spatial (Salmina y Filomonova, 2002; Solovieva, Ortiz y Quintanar, 2010; Solovieva, Quintanar & Ortiz, 2012).
The objective of our study consisted in implementation of methodology of formative experiment and gradual formation of scientific concepts for the theme of decimal concept in primary school. The work was fulfilled by post graduated student of Master in Neuropsychology of Faculty of Psychology of Autonomous University of Puebla. The work represents a part of Master Dissertation of this institution, elaborated and carried out together with professors of the Program.
Method
Participants
Children of second grade of elementary school were included in formative experiment. The average age of the children was 7.25 years. The requirements to be able to participate in the experiment were: Being in 2ndgrade of elementary school for the first time, and do not present neurologic background. The table 1 shows the characteristics of the participants.
Table 1. Description of the participants
|
Subjetc |
Age |
Gender |
Laterality |
|
A |
7 |
M |
Lefthanded |
|
B |
8 |
M |
Righthanded |
|
C |
7 |
F |
Righthanded |
|
D |
7 |
M |
Righthanded |
Workspace
The experiment took place in a small new private college, located in the city of Puebla, Mexico. The figure 1 represents the workspace.

Material
The protocol “Verification del exito escolar en la escuela primaria” (Solovieva y Quintanar, 2003), based on Luria's conception of High Psychological Functions, was applied. Also, tasks of measure comparison, arithmetic problems resolution, addition and subtraction were included. The figure 2 presents some examples of the initial evaluation test.


The same tasks were applied in the final evaluation and others with higher complexity, some examples are shown in the figure 3.

Experiment
A formative experiment concept was used according to the authors of historical and cultural psychology (Talizina, 1998; Salmina, 2001, Talizina, Solovieva y Quintanar, 2010). In such studies the method consists of gradual formation of concepts, skills or actions starting from level “0”, that is, providing gradual cooperation within the zone of proximate development, according to L.S. Vigotsky’s conception. The formation is organized and guided by an adult who knows the operative structure and all kinds of necessary orientation which can guarantee reflective and conscious acquisition of proposed concepts. At the end such skills are tested in common and new tasks which are proposed to children.
At the same time an experimental design was used.
G1: O1 X O2
G1= experimental grup; O1= Pretest; X= teaching method; O2= Postest.
Procedure
The divided collective activity in groups and in pairs of children was considered for all steps of the program. The actions with measuring of magnitudes and work with different objects were included. The program includes specific tasks for symbolic, logical, numeric and spatial component. The Program was applied 3 days per week during six months. In the following table (2) the thematic, objectives, and materials of the teaching method are shown.
Table 2. Tasks of teaching method
|
Subject |
Task |
Objectives |
Stage |
Materials |
|||
|
Measure |
a) Object measuring b) Distance measuring |
a) Make students identify the measure components and perform measurements: Magnitude (M), measure (m), and quantity of times the measurement was used (v). |
M O T I V A T |
B O A |
C O N T R O |
Material Materialized Perceptive |
-Commonly used objects by children -Drawn animals -Drawn paths - Measurement orientation card - Measurement |
|
|
|
|
I O N |
|
L |
|
scheme |
|
Teaching of unit |
a) Object measuring b) Distance measuring c)Comparison measurements: Less than (<), greater than, (>) and equal (=) |
a) Make students identify one common measurement (unit) to compare objects. |
Materialized Perceptive |
-Drawn animals -Drawn paths -Ribbons |
|||
|
Teaching of ten |
a) Object measuring b) Distance measuring c)Comparison measurements: Less than (<), greater than, (>) and equal (=) d) Table preparation degrees |
a) Make students identify a ten as a new measurement. b) Make student identify the relationship between different units of the numeric system. |
Materialized Perceptive External speech |
-Positional value table of the numeric system -Matches -Drawing of objects |
Below the teaching method and some examples are presented.
Subject 1. Measure: Measurements were performed considering various objects and magnitudes. Specific concepts like “Magnitude”, “Measure” and “Number” were introduced and explained to children form the very beginning (the figure 4 shows the orientation card for this task). In this case, “Number” corresponds to the quantity of times a measurement is used. These elements are described in the card "Measure", where children had to use this card for every concrete example. Firstly, they chose an object and a feature of an object to measure (e.g. a highness or broadness of a toy, window, board, distance between two places in the classroom or between tow children, water bottles) and something with which we can to measure such objects (pencils, chalks, paper strips, glasses and so on). The measurement of volumes of different liquids with the help of specific measures was used as well. Subsequently, the gathered data was written down on the students notebooks using the "Measurement Scheme". The Figure 5 shows an example of a task of the materialized stage, in which a drawn path is measured using a paper foot.


Subject 2. Teaching of unit: In this stage, every task was performed using a common measurement to compare different objects and liquids. In this case, the size to be measured was chosen by all the participants (the same ribbon).The goal was to teach children that the smaller was the size of the measurement, the more times they would have to use it, and the other way around, the bigger was size of the measurement the lesser times they would have to use it. The Figure 6 shows how our children measured distances (“Magnitude”) between various objects or places. In some exercises children had find out which roads where longer or shorter. Matches were used by children to represent the quantity of times the measurement (a ribbon) was used. All data was recorded on the board and a comparison was made between the paths and lines in all occasions. The children used mathematical signs “less than” (<), “greater than” (>) and “equal” (=) to understand the relationships between the magnitudes worked. For each answer they chose the appropriate symbol with the help of the adult.

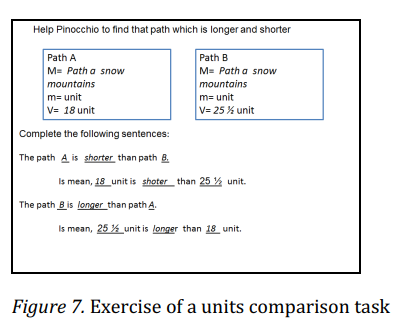
The Figure 7 shows an example of a paths comparison exercise in the perceptual stage. The children are given a sheet with two paths drawn and they are asked to measure them using a ribbon. Afterwards, the students write down the data obtained in a registry sheet and identify the measurement used, the shortest path, the longest path and their numeric representation.
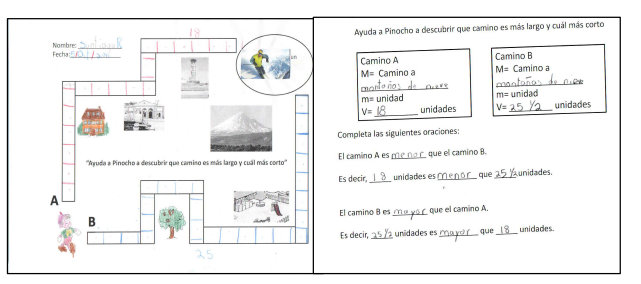
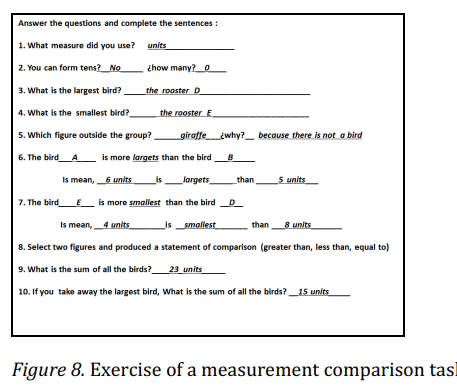
Afterwards the students worked with a number of images that indicated the quantity of times a measurement was used. The goal of this exercise was to answer a variety of questions focused on the identification of the measurement and the semantic group, the comparison between objects (lesser than, greater than or equal), and the numeric representation of these comparisons. The figure 8 shows an example measuring the size of different birds and the questions used for this exercise.

The Figure 9 shows an example of liquids measurement using a magnitude of a water bottle. The measurement was a small cup used by the boy and wooden sticks were used to represent how many times the cup was used.

Subject 3. Teaching of ten (NS). The ten was introduced to children as a new unit of measurement. The children were taught that this new unit was obtained by adding up 10 units together and is placed on the left side of the units. Figure 10 show some example of tens localization.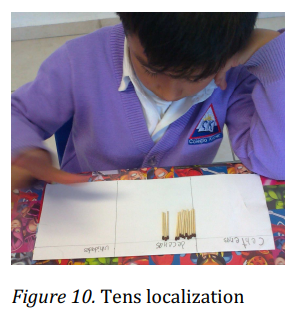
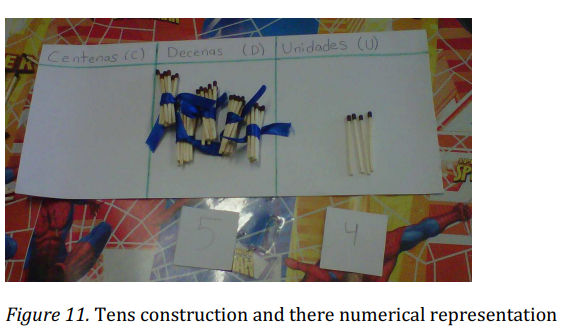
Afterwards, children joined groups of ten matches together using a ribbon to tie them up, these groups of matches represented the tens and were used to build up other units of measurements (hundreds and thousands). The figure 11 shows an example of tens constructions and their localization on the positional value table.
After having finished the materialized stage the children were moved onto the perceptive stage. This stage goal is to identify the units of measurement used in a number of images and put them into groups. Figure 12 demonstrates an example.
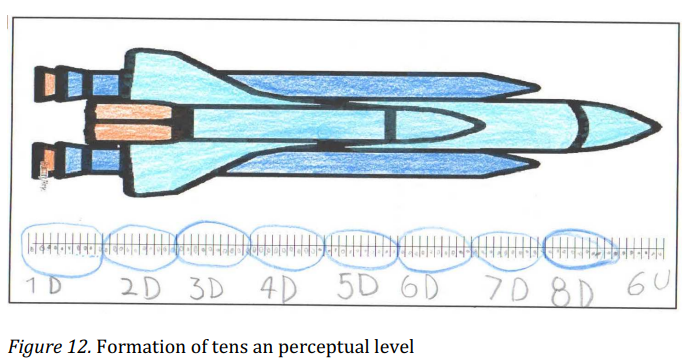
Figure 13 shows the data record used for the previous task and the questions they had to answer about the identified units.

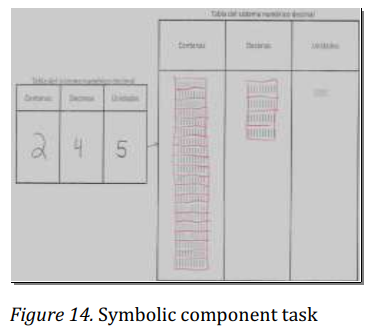
The next step was to teach children how to get a numeric representation based on groups of tens represented in a graphical way using the positional value table as shown in figure 14.
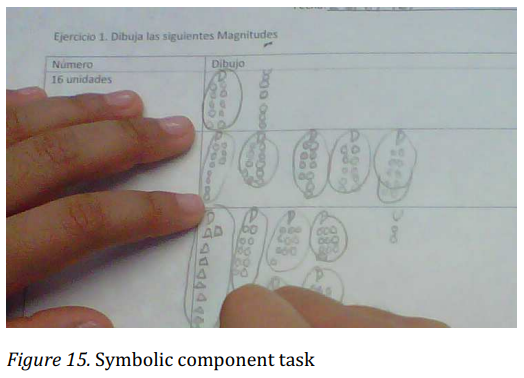
After children were able to identify the units of measurement out of a picture they were given the opposite task. They had to make a graphical representation of a number using the positional value table. Figure 15 shows an example.
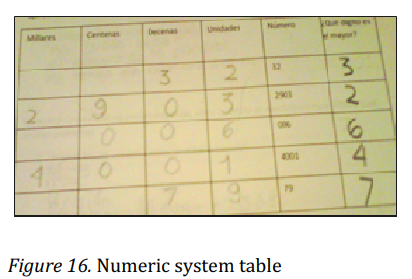
The final task consisted on the localization of different numbers using the numeric system table, and the identification of the biggest and the smallest digit on them. Figure 16 shows an example.
Results
Before and after implementing the program a qualitative analysis of the mathematical skills and components was performed. The initial evaluation demonstrated that children had not developed any general skills to assimilate the concept of decimal numeric system, actually, most of the tasks were almost impossible to be performer by the students unless they used back up material. In the final evaluation these problems were overcome by them, and they were able to finish all the tasks independently (only one student required perceptive support). Table 3 shows the evaluations results.
Table 3. Types of error in Pretest-Postest
|
Area evaluated |
Pretest |
Type of support |
Postest |
Type of support |
|
|
Mathema tical compone nts |
Special Compon ent |
- Difficulty in identify the biggest and smallest digit in the number |
Executi on impossi ble (A,B,C,D ) |
- Identify the biggest and smallest digit in the number |
Indepen dent executi on (A,B,C, D) |
|
Symboli c Compon ent |
- Difficulty transcription numerical language problem - Difficulty in name conjunction with the name of the figure and its inverse (reversibility) |
Executi on impossi ble (A,B,C,D ) |
- Transcription numerical language problem - Name of the conjunct and its inverse (reversibility) |
Indepen dent executi on (A,B,C, D) |
|
|
Logic Compon ent |
- Difficulty of data relation the problems - Difficulty in conserving the |
Stage materia l (A,B,C,D |
- Data relation the problems - Conserving the amount |
Indepen dent executi on (A,C, D) |
|
|
|
|
amount |
) |
|
Stage Percept ual (B) |
|
Mathem atic Compon ent |
- Difficulty in identify the measure - Difficulty in the next units of measurement |
Executi on impossi ble (A,B,C,D ) |
- Identify the next units of measurement |
Indepen dent executi on (A, B, C, D) |
The results obtained during the implementation of the teaching method are shown in Table 4, the number of exercise worked, the level of execution and types of errors. Only two mistakes were made and the beginning of the method.
According to final results no systemic difficulties have taken place. Children solved most of the tasks independently. If children had any kind of difficulty, they asked for help immediately. The level of external assistance was verbal, in contrast to the initial assessment in which the level of external help was always at level of concrete actions. Three students (A, C, D) were able to perform tasks in the verbal and independent external level. The other student (B) required support in the perceptive stage (see figure 17).
Table 4. Tasks used in the teaching method
|
Subject |
Stage |
Task Number |
Correct |
Incorrect |
Type of error |
|
Measure |
Material- Materializad |
84 |
82 |
2 |
Difficulty in identifying the measure |
|
Teaching Units |
Perceptual |
81 |
81 |
0 |
|
|
Teaching Ten |
Perceptual External Verbal |
194 |
194 |
0 |
|

In table 5 the answer given by student (A) before and after the method application are shown.
Table 5. Measure task comparison
|
Task |
Initial evaluation test |
Final evaluation test. |
|
Which one is bigger, 3 |
3, because is bigger than |
1 meter is bigger than 3 centimeters because 1 |
|
cm o 1 m? |
one. |
meter has 100 centimeters |
|
Which one is bigger, 5 liters or 2 kilograms? |
5, because is bigger than two. |
Is not possible they are different measurements |
|
Which one is bigger, two quarters of hour or a half hour? |
I don't know, i haven’t been taught to read the clock. |
Its equal because one quarter plus one quarter equals a half hour |
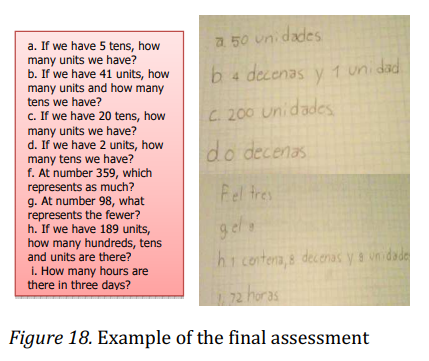
The final assessment has shown that the children were able to identify the positional value of digits in a number, to write the number correctly, to compare measures correctly, to perform arithmetic operations and to understand relationships in the decimal numeric system. Figure 17 shows examples of the final evaluation. The children performed the proposed tasks by themselves at the verbal level. Such result had not been observed before working with the program.
Discussion and conclusions
We emphasize that teaching should be understood as directed and organized process as proposed by the Activity Theory (Talizina, Solovieva and Quintanar, 2010) and not as empiric configuration of various operations and actions. The teacher should clearly understand what is he/she teaching and how the best result could be achieved. Such organization of teaching process allows the children to be conscious of their learning. Only in this case the teaching process could be based on learning motive. The design of phased program of training mental actions (Talizina, 2009; Galperin, 1969) allows and guarantees the development of mathematical skills in our children. In this research we show how the work on the first stage of teaching process stage was organized with inclusion the actions of measurement of features of objects (e.g. highness, broadness and volume) by children with constant orientation and participation of an adult. Only such actions permit to guarantee successful assimilation and generalization of the concept of decimal number system (as proposed by P.Ya. Galperin and continued by his followers). This action is essential for children to assimilate the concept of decimal number system and arithmetic operations (Dadidov, 1988; Salmina, 2010).
After such training, there were no errors in reading of new numbers displayed for the children as some researchers have found as constant negative feature of children at primary school in different countries (Butto y Gomez, 2011; Garcia, 2011: Gomez, 1991).
The principles of activity theory allow to create systems of strategy for actions that ensure the understanding of mathematical concepts, as opposed to the constructivist methods of working just with separate individual actions of children with no specific purpose established and putting more emphasis on the teaching of symbols just as any other external object in front of the child (Martin, 2003; Castano, 2008). The techniques used in constructivist approach never retake the measurement action properly. Such methods pretend to measure only empirically without any objective. The measurement is presented as a kind of “free individual” process for independent “construction” of the knowledge by each child without any orientation. Such approach is very common and dominants the system of teaching of mathematic abilities in may countries.
The concept of the decimal numeral system involves the usage of measurement and the relationships that emerge from it (Salmina, 2001). Actually, various materials for teaching the academic content exist, among them which Cuissner strips, Bancubi method, Abacus, figurative, numerical bands and tied. However, these techniques use the symbol from the start as one of the objects which need to be counted, so that the children and can not see any relationship between the numbering system, the number and the symbol (Silva & Barela, 2010). No real relation between action of measure and usage of symbols is established in these proposals. Children use symbols as “objects” and never discover the logic or symbolic sense of their operations. We can even say that they use “symbols” as external objects which they count and not as “symbols”. The precise content of symbols is never presented to the children. In such situation, children commonly, if not always, show strong difficulties in mathematics up to secondary school (two of every five Mexican students 15 years, 39% is located in the lower levels of performance, OCDE, 2012) and absence of comprehension of the symbolic and abstract nature of the concept of number (Solovieva, Ortiz & Quintanar, 2010).
We hope that that our future research could work with significant samples for generalization of the results of our data. It is also possible to continue proposes of teaching and monitoring the decimal number system by introduction of fractions, which could be managed as a results of action of division and not as part of the measure as some authors propose (Block & Solares, 2001). We conclude that the formation of decimal numeral system concept depends on the formation of actions such as measuring, comparing, classifying, logical, spatial and directed teaching (Salmina, 2001).
References
- Ávila, A. y García, S. (2008). Los decimales: más que una escritura. México: INEE.
- Block, D. y Solares, D. (2001). Las fracciones y división en la escuela primaria: análisis didáctico de un vínculo. Educación matemática, 13 (2).
- Butto, C. y Gómez, L. M. (2011). Las representaciones del sistema numérico decimal indo-arábigo en niños de primer grado de primaria. XIX Congreso Mexicano de Psicología. Cancún Center, Cancún, Quintana Roo, México.
- Castaño, J. (2008). Una aproximación al proceso de comprensión de los numerales por parte de los niños: relaciones entre representaciones mentales y semióticas. Univ. Psichol., 895-907.
- Davídov, V. (1988). La enseñanza escolar y el desarrollo psíquico. Moscú: Progreso.
- Galperin, P. Ya. (2009). La formación de los conceptos y de las acciones mentales. En Quintanar, L. y Solovieva, Y. (2009) (Comps.). Las funciones psicológicas en el desarrollo del niño. México: Trillas.
- García, O. (2011). Las matemáticas naturales del niño y su transición a la educación formal en la escuela formal. XIX Congreso Mexicano de Psicología. Cancún Center, Cancún, Quintana Roo, México.
- Gómez, C. (1991). Cognición, contexto y enseñanza de las matemáticas. Comunicación, lenguaje y educación, 11, 11-26.
- Martín, A. (2003). Operación aritmética: la división. Boletín: Las matemáticas en secundaria, 25.
- OCDE (2012). Perspectivas México: Reformas para el cambio. México: OCDE.
- Ruesga y Guimaraes, (2011). Sistema de numeración decimal: un instrumento para seleccionar libros de texto de los tres primeros años de enseñanza. Indicações para os trabalhos da, 13, pp 1-12.
- Salmina, N. (2001). La enseñanza de las matemáticas en la escuela primaria. En Talizina, N. (2001). La formación de las habilidades del pensamiento matemático. México: Universidad Autónoma de San Luis Potosí
- Salmina, N. y Filiminova, O. (2002). Problemas en el aprendizaje de las matemáticas básicas y su corrección. Moscú: Instituto universitario de estudios avanzados.
- SEP (2011). Evaluación Nacional de Logro Academico en Centros Escolares, ENLACE. México: SEP.
- Silva, A. y Barela, C. (2010). Los materiales concretos en la enseñanza de la numeración. Quehacer educativo, pp 26-33.
- Solovieva, Ortiz y Quintanar (2010). Formación de conceptos numéricos iniciales en una población de niños mexicanos. Cultura y educación, 20 (2).
- Solovieva, Y. y Quintanar, L. (2010). El desarrollo del niño y los métodos de enseñanza. Elementos, 77, 9-13.
- Solovieva Yu., Quintanar L. & Ortiz, G. (2012). The Formation of Initial Components of Number Concepts in Mexican Children. Psychology Research, 2 (7), 422-430.
- Talizina, N. (2009) La teoría de la actividad aplicada a la enseñanza. México: BUAP.
- Talizina, T., Solovieva, Y. y Quintanar, L. (2010). La aproximación de la actividad en psicología y su relación con el enfoque histórico-cultural de L. S. Vygotsky. Novedades educativas, 230, pp. 4-8.
Information About the Authors
Metrics
Web Views
Whole time: 1678
Previous month: 3
Current month: 1
PDF Downloads
Whole time: 781
Previous month: 3
Current month: 0
Total
Whole time: 2459
Previous month: 6
Current month: 1